网络重构最新综述推荐:从宏观尺度到微观尺度

导语
如何利用部分网络数据推断网络的宏观属性?能否进一步推断出网络中隐藏的链接模式,乃至补全中的具体节点与连边?最近arXiv的一篇综述文章,以统计物理和信息理论的视角,从宏观、介观和微观三个尺度讨论了解决网络重构问题的思路、方法和技术,本文是对综述整体内容的介绍。综述原文106页,扫描下方二维码即可查看下载PDF文件。


陈昊 | 作者
刘培源 | 审校
邓一雪 | 编辑
论文题目:
Reconstructing networks
论文地址:
https://arxiv.org/abs/2012.02677
1. 宏观尺度的网络重构
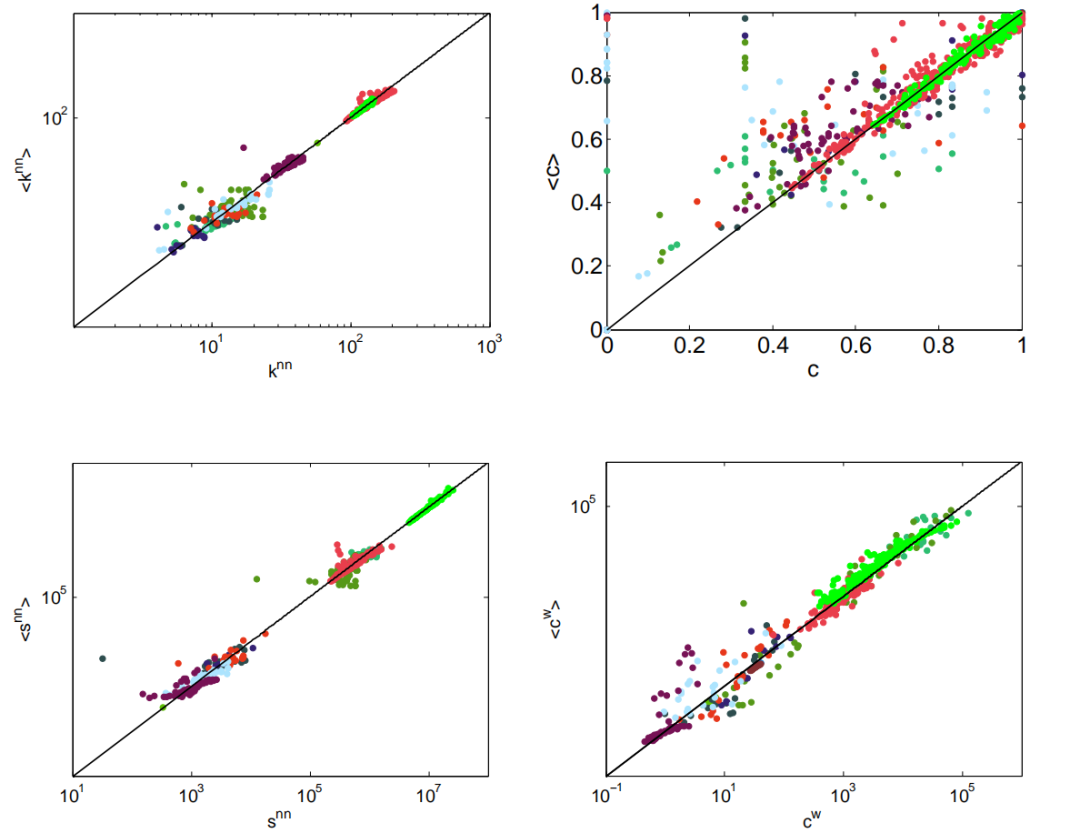
图1. 已知 srengths 和度的情况下,ECM 模型的表现。每张子图都展示了针对某些网络实际节点特定网络属性的重构结果(Y轴)与理论值(X轴)左上:最近邻居平均度(average nearest neighbors degree),右上:集聚系数(clustering coefficient) ,左下:最近邻居平均强度(average nearest neighbors strength) ,右下:加权聚类系数(weighted clustering coefficient)[3]
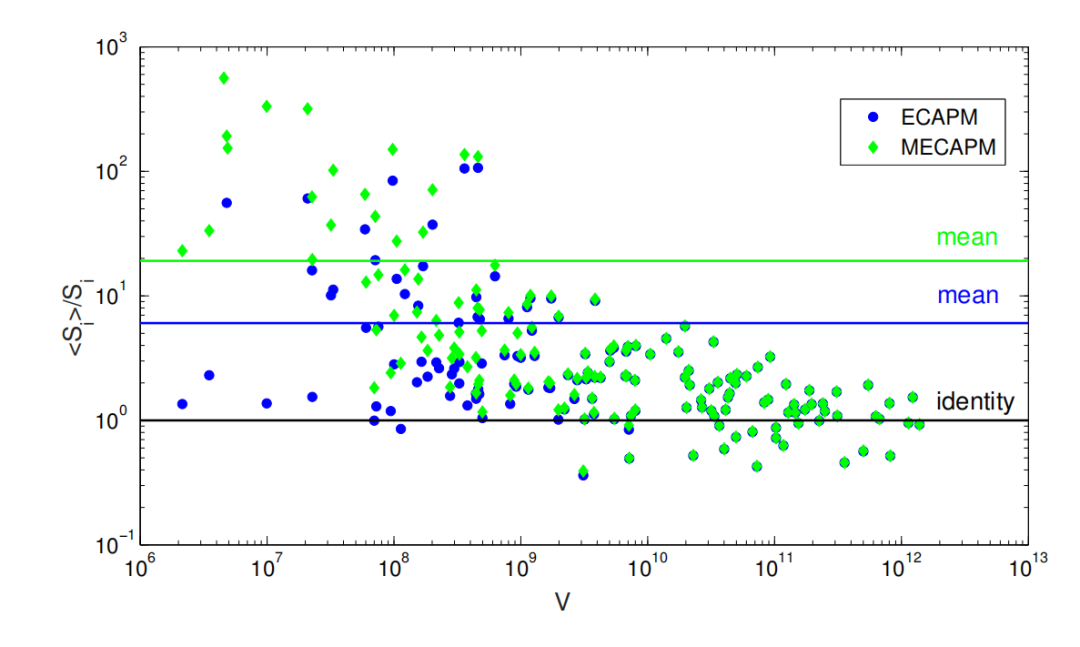
图2. 在 SHS 网络数据集中重构网络系统性:每个点代表一个具体部门使用dcGM(蓝色)或MECAPM(绿色)推断出的相对系统性,两种方法的平均值均以水平实线表示。[14]
2. 介观尺度的网络重构
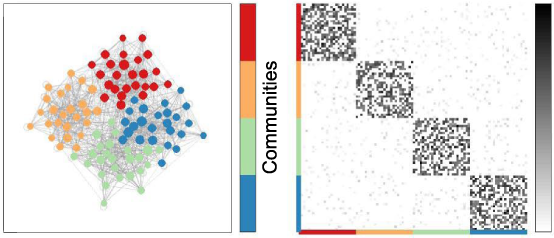
2.1 社区结构
2.2 核心-边缘结构
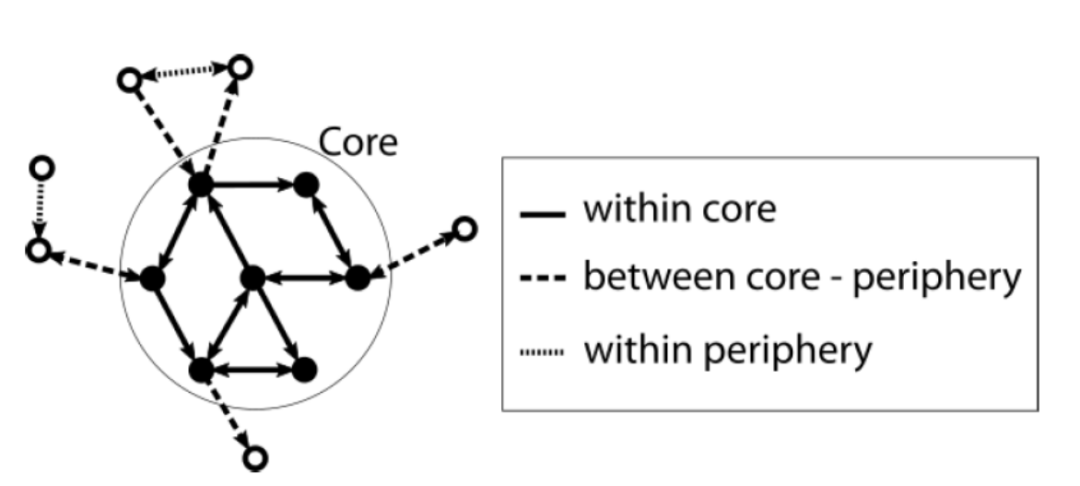
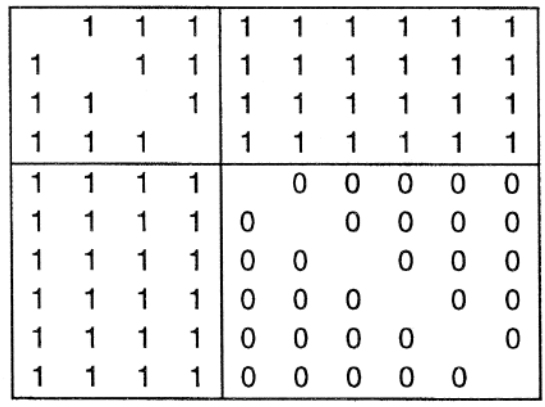
图4. 核心-边缘结构网络示例 左图为核心-边缘结构网络中的节点类型,右图为严格符合核心边缘结构的网络的邻接矩阵[6]
3. 微观尺度的网络重构
3.1 基于相似度的算法
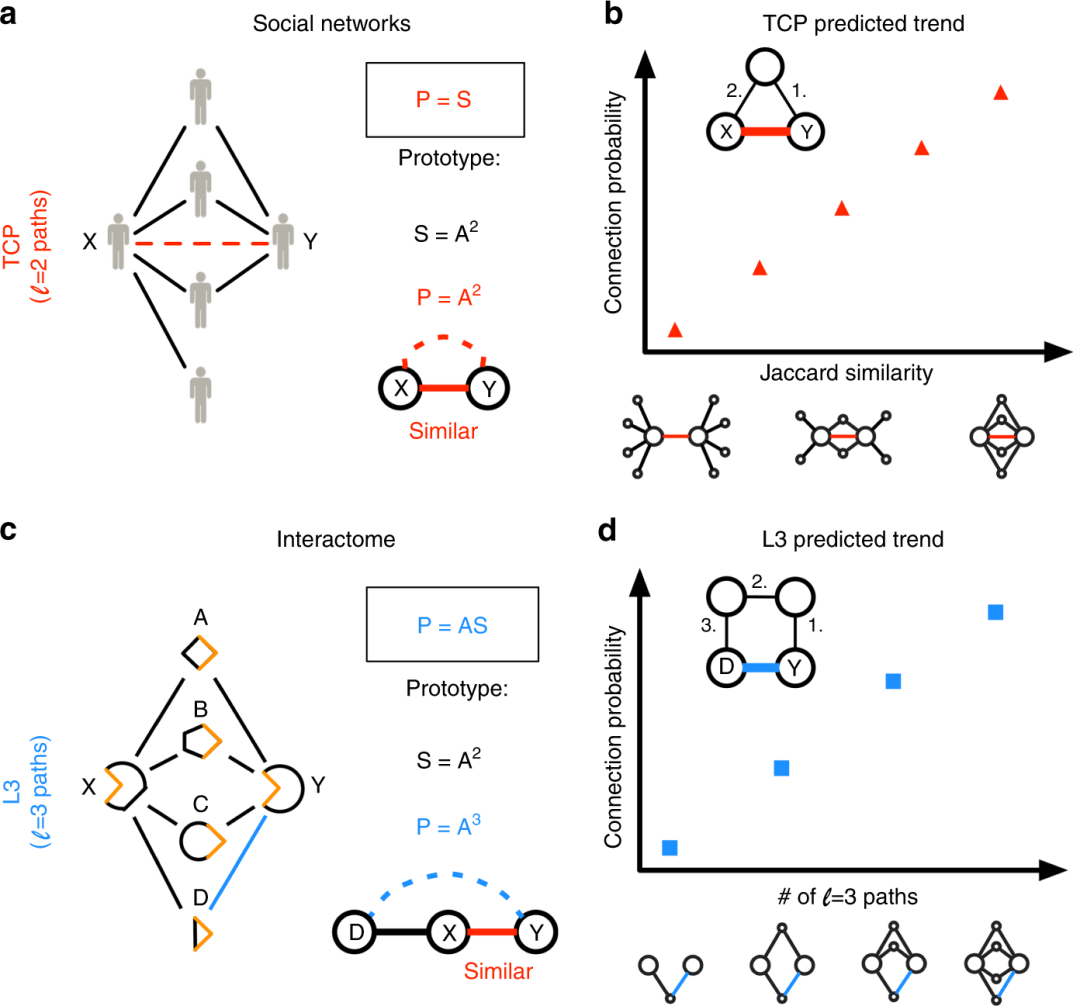
图5. 社交网络与蛋白质网络中的链路预测 图a为社交网络中连边产生的示意图。图b为在满足三元闭包原理(Triadic Closure Principle)的网络模型下不同状态的节点对间产生连边的概率。图c为蛋白质相互作用中连边产生的示意图。图d为在满足四元闭包原理(Quadrangular Closure Principle)的网络模型下不同状态的节点对间产生连边的概率。[7]
3.2 基于模型的算法
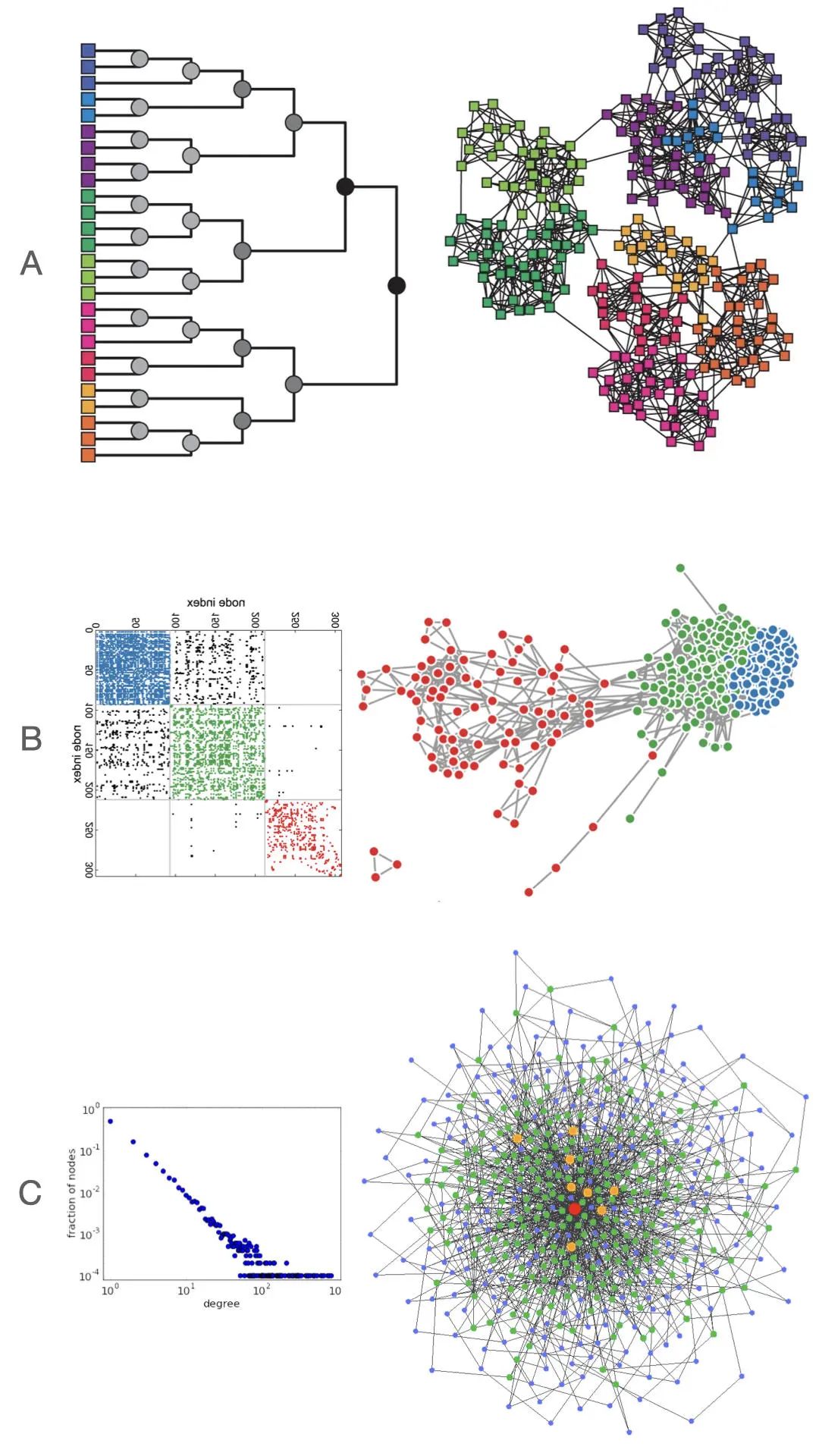
图6. 在基于模型的算法上对网络结构进行采样 (A)分层结构网络 (B)块状结构网络 (C)无标度网络[8-10]
4. 小结
[1] Squartini, Tiziano and D. Garlaschelli. “Analytical maximum-likelihood method to detect patterns in real networks.” New Journal of Physics 13 (2011): 083001.
[2] Gangi, Domenico Di et al. “Assessing Systemic Risk Due to Fire Sales Spillover Through Maximum Entropy Network Reconstruction.” Regulation of Financial Institutions eJournal (2018): n. pag.
[3] Mastrandrea, R. et al. “Enhanced reconstruction of weighted networks from strengths and degrees.” New Journal of Physics 16 (2014): 043022.
[4] Cimini, G. et al. “Systemic Risk Analysis on Reconstructed Economic and Financial Networks.” Scientific Reports 5 (2015): n. pag.
[5] Faskowitz, J. et al. “Weighted Stochastic Block Models of the Human Connectome across the Life Span.” Scientific Reports 8 (2018): n. pag.
[6] Borgatti, S. and M. Everett. “Models of core/periphery structures.” Soc. Networks 21 (2000): 375-395.
[7] Kovács, István A. et al. “Network-based prediction of protein interactions.” Nature Communications 10 (2019): n. pag.
[8] Clauset, A. et al. “Hierarchical structure and the prediction of missing links in networks.” Nature 453 (2008): 98-101.
[9] Larremore, D. et al. “A Network Approach to Analyzing Highly Recombinant Malaria Parasite Genes.” PLoS Computational Biology 9 (2013): n. pag.
[10] Oikonomou, Panos and P. Cluzel. “Effects of topology on network evolution.” Nature Physics 2 (2006): 532-536.
[11] Alon, U.. “Network motifs: theory and experimental approaches.” Nature Reviews Genetics 8 (2007): 450-461.
[12] Leskovec, J. et al. “Predicting positive and negative links in online social networks.” WWW ’10 (2010).
[13] Tylenda, Tomasz et al. “Towards time-aware link prediction in evolving social networks.” SNA-KDD ’09 (2009).
[14] Squartini, Tiziano et al. “Enhanced capital-asset pricing model for the reconstruction of bipartite financial networks.” Physical review. E 96 3-1 (2017): 032315 .
[15] Holme, P.. “Core-periphery organization of complex networks.” Physical review. E, Statistical, nonlinear, and soft matter physics 72 4 Pt 2 (2005): 046111 .
[16] Rossa, F. et al. “Profiling core-periphery network structure by random walkers.” Scientific Reports 3 (2013): n. pag.
[17] Jeude, J. V. L. D. et al. “Detecting Core-Periphery Structures by Surprise.” ArXiv abs/1810.04717 (2018): n. pag.
课程推荐

复杂科学最新论文
集智斑图顶刊论文速递栏目上线以来,持续收录来自Nature、Science等顶刊的最新论文,追踪复杂系统、网络科学、计算社会科学等领域的前沿进展。现在正式推出订阅功能,每周通过微信服务号「集智斑图」推送论文信息。扫描下方二维码即可一键订阅:


推荐阅读
点击“阅读原文”,追踪复杂科学顶刊论文