复杂系统的子采样问题：如何从部分推断整体涌现特性？

导语

复杂系统往往包含大量相互作用的单元，如何通过观测部分单元的数据来了解整体的涌现特性？如何结合不同时间和空间尺度的数据以全面理解复杂系统？近日发表于 Nature Reviews Physics 的一项题为“处理子采样问题从有限数据中推断集体属性”的新研究指出，统计物理中的标度理论可以用来解决这一子采样问题。

关键词：复杂系统，标度理论，统计物理，涌现

潘佳栋 | 作者

梁金 | 审校

邓一雪 | 编辑

原文题目：

Tackling the subsampling problem to infer collective properties from limited data

原文链接：

https://www.nature.com/articles/s42254-022-00532-5

复杂系统是迷人的，因为它们丰富的宏观特性是由许多简单的部分相互作用而产生的。了解自然界中这些涌现现象，需要通过实验来评估自然复杂系统。然而，尽管人们发展了大规模的数据采集技术，但是实验观察往往只限于系统的一小部分。这种空间子采样在神经科学中尤为严重，在那里只有几百万甚至几十亿的神经元的极小部分可以被单独记录。

当从子采样的部分推断整个系统的集体属性时，空间子采样可能导致重大的系统偏差。为了克服这种偏差，人们开发了强大的数学工具。在这篇文章中，作者概述了一些由子采样产生的问题，并回顾了最近开发的解决子采样问题的方法。这些方法使人们能够通过观察系统的一小部分来正确评估，例如图的结构、动物的集体动力学、神经网络活动或疾病的传播。最后概述了子采样问题的主要开放性挑战。在解决这些挑战的同时，大规模记录技术的发展将使人们对复杂的生命系统的工作有进一步的基本了解。

1. 复杂系统的子采样问题

复杂系统，特别是生命系统，是由众多具有不同相互作用的单元组成的：粒子在碰撞，细胞在生长、运动和分裂，神经元在远距离发送信号。这些相互作用产生了一系列涌现现象[1]，包括模式形成[2-6]、同步[7、8]、集群[9、10]、渗流[11-13]和自组织临界[14-20]。了解这些涌现现象及其起源对于理解、预测和影响复杂系统是必要的[21]。

图1：子采样的分类。a-c：空间嵌入网络典型结构示意图；d-f：从空间扩展系统中采样的三种方法。随机采样(d)的优点是绘制一组具有代表性的节点。加窗采样(e)提供了良好的局部分辨率，但样本可能不能代表整个系统；滚雪球采样(f)跟踪选定节点的链接，从而揭示该节点的连通性。

然而，在很多人们感兴趣的复杂系统中，研究人员无法通过简单地以全分辨率观察所有的单元来实现这一目标，因为单元数量太多了。因此，在实验中，人们必须依靠粗略采样或空间子采样。在这两种情况下，采样方式的选择都会对观测数据产生强烈的影响，甚至产生偏差，因此，对理解集体特性提出了挑战[22-27]。

当进行粗略采样，也就是以粗略的分辨率对整个系统进行采样时，人们基本上可以直接评估系统的大尺度涌现特性。然而，这种粗略的、间接的测量只提供了宏观的“观测值”，可能无法充分了解单元之间的微观互动[28,29]，而这种互动对于解释涌现的复杂现象是至关重要的。

研究人员因此面临一个挑战，即利用时间和空间上的精确测量来填补这些缺失的微观参数，然而，这些测量只能作为子样本观测。因此，有必要了解有限采样带来的挑战，然后弥合采样和完整系统之间的差距，以实现对涌现现象的完整理解。

2. 标度理论克服子采样难题

在统计物理学的基础上，克服子采样问题的一个直观方法是采用有限尺度标度理论（finite-size scaling）的方法。有限尺度标度理论被开发出来用于研究复杂系统的尺度不变性。这种标度理论的一个常见方法是制定一个多变量函数，描述系统属性对不同参数的依赖性；确定这些参数的关联尺度；并对这些参数进行重新标度，使其产生一个普适的函数，不再依赖于基础系统的微观细节。对于具有尺度不变性的系统来说，这种转换预计将由所谓的临界指数来描述。这种标度理论可以自然地被扩展以克服子采样问题。

图2：标度理论在加窗采样时可以揭示集体动力学的宏观性质。a. 局部连接的二维网络中的加窗采样示意图。b. 从尺寸W的人工子采样窗口中的空间关联长度r*的标度，可以评估系统的种群动态是否接近临界值。c. 最近针对神经元活动提出了一种互补的启发式粗粒化方案，其潜在联系未知。d. 不同聚类大小 K 的归一化特征值的标度揭示了临界行为的特征。

作者概述了从子采样研究复杂系统集体性质的新技术。这些新方法的发展在很大程度上是由实验技术的快速发展推动的，这些实验技术允许更好地（但远非完全）逼近自然复杂系统。这种发展是否意味着人们可以简单地等到采样技术足够好的时候再使用给定的分析方法？当然不是！尽管实验技术不断进步，但由于技术原因，可能总会有系统无法完全采样。对于大型神经系统，如哺乳动物的大脑，目前尚不清楚如何在不损害脑组织的情况下记录大脑深处区域所有神经元的活动。因此，补充现有分析方法以尽可能多地从子采样数据中学习仍然是当前的研究热点。

3. 多尺度采样帮助全面理解复杂系统

对复杂系统的理解可以从不同尺度的联合观测中获益匪浅。对多尺度测量的综合评估确实是一个艰巨的挑战，但它有望以一种协同的方式克服每个尺度采样的限制，从而有力地改善对整个系统的多尺度活动的推断。一个有希望的研究途径是改进数学工具集，将这些不完美但互补的记录技术的数据结合起来。在理论层面上，为加窗和随机子采样开发一个统一的理论可能需要一个新的标度理论，但如果成功，可以大大推进对系统行为的准确评估。因此，考虑不同采样技术的组合可能会有助于评估系统行为，因为有趣的涌现行为通常是在多个尺度上演变的。

一个重要但尚未解决的问题是，如何结合不同时间尺度的样本。特别是对于不断变化的生命系统，这样做绝不是一个微不足道的问题。以大脑为例，通过监测同一神经元的重复任务，表明神经表征可能随时间的推移而发生变化。然而，在许多系统中，实验的限制可能不允许同时访问多个空间尺度，而只是连续地访问。这种限制可能会阻碍对微观尺度的相互作用如何影响宏观尺度的涌现行为的理解，反之亦然。整合在不同空间尺度上获取的信息，即使不是平行记录，也是一个进一步的开放性挑战。然而，作者期望在非平衡物理学领域做出重要贡献，在控制良好但非稳态的系统中描述子样本的跨时间和空间尺度的影响。

总的来说，最近采样技术的发展与系统分析方法一起，预示着空间子采样理论将变得更加广泛，而且随着数据可用性的增加，也会变得更加强大。这就需要未来的研究来解决问题，特别是如何设计创新的采样策略（即如何选择被采样的部分），如何处理一般的非代表性样本，如何结合不同类型的空间子样本（例如通过多尺度采样），以及如何设计因果干预措施。

参考文献

Muñoz, M. A. Colloquium: Criticality and dynamical scaling in living systems. Rev. Mod. Phys. 90, 031001 (2018).
Cross, M. C. & Hohenberg, P. C. Pattern formation outside of equilibrium. Rev. Mod. Phys. 65, 851–1112 (1993).
Gollub, J. P. & Langer, J. S. Pattern formation in nonequilibrium physics. Rev. Mod. Phys. 71, S396–S403 (1999).
Cross, M. & Greenside, H. Pattern Formation and Dynamics in Nonequilibrium Systems 1st edn (Cambridge Univ. Press, 2009).
Desai, R. C. & Kapral, R. Dynamics of Self-Organized and Self-Assembled Structures (Cambridge Univ. Press, 2009).
Halatek, J. & Frey, E. Rethinking pattern formation in reaction–diffusion systems. Nat. Phys. 14, 507–514 (2018).
Acebrón, J. A., Bonilla, L. L., Pérez Vicente, C. J., Ritort, F. & Spigler, R. The Kuramoto model: a simple paradigm for synchronization phenomena. Rev. Mod. Phys. 77, 137–185 (2005).
Arenas, A., Díaz-Guilera, A., Kurths, J., Moreno, Y. & Zhou, C. Synchronization in complex networks. Phys. Rep. 469, 93–153 (2008).
Cavagna, A., Giardina, I. & Grigera, T. S. The physics of flocking: correlation as a compass from experiments to theory. Phys. Rep. 728, 1–62 (2018).
Rahmani, P., Peruani, F. & Romanczuk, P. Topological flocking models in spatially heterogeneous environments. Commun. Phys. 4, 206 (2021).
Christensen, K. & Moloney, N. R. Complexity and Criticality (World Scientific, 2005). 12. Araújo, N., Grassberger, P., Kahng, B., Schrenk, K. & Ziff, R. Recent advances and open challenges in percolation. Eur. Phys. J. Spec. Top. 223, 2307–2321 (2014).
Korchinski, D. J., Orlandi, J. G., Son, S.-W. & Davidsen, J. Criticality in spreading processes without timescale separation and the critical brain hypothesis. Phys. Rev. X 11, 021059 (2021).
Bak, P., Tang, C. & Wiesenfeld, K. Self-organized criticality: an explanation of the 1/f noise. Phys. Rev. Lett. 59, 381–384 (1987).
Jensen, H. J. Self-Organized Criticality: Emergent Complex Behavior in Physical and Biological Systems (Cambridge Univ. Press, 1998).
Dickman, R., Munoz, M. A., Vespignani, A. & Zapperi, S. Paths to self-organized criticality. Braz. J. Phys. 30, 27–41 (2000).
Aschwanden, M. Self-Organized Criticality in Astrophysics: The Statistics of Nonlinear Processes in the Universe (Springer, 2011).
Pruessner, G. Self-Organised Criticality: Theory, Models and Characterisation (Cambridge Univ. Press, 2012).
Hesse, J. & Gross, T. Self-organized criticality as a fundamental property of neural systems. Front. Syst. Neurosci. 8, 166 (2014).
Zeraati, R., Priesemann, V. & Levina, A. Self-organization toward criticality by synaptic plasticity. Front. Phys. 9, 619661 (2021).
Boccaletti, S., Latora, V., Moreno, Y., Chavez, M. & Hwang, D. U. Complex networks: structure and dynamics. Phys. Rep. 424, 175–308 (2006).
Stumpf, M. P. H., Wiuf, C. & May, R. M. Subnets of scale-free networks are not scale-free: sampling properties of networks. Proc. Natl Acad. Sci. USA 102, 4221–4224 (2005).
Serafino, M. et al. True scale-free networks hidden by finite size effects. Proc. Natl Acad. Sci. USA 118, e2013825118 (2021).
Morstatter, F., Pfeffer, J., Liu, H. & Carley, K. M. Is the sample good enough? Comparing data from Twitter’s Streaming API with Twitter’s Firehose. Proc. Int. AAAI Conf. Weblogs Soc. Media 7, 400–408 (2013).
Priesemann, V., Munk, M. H. & Wibral, M. Subsampling effects in neuronal avalanche distributions recorded in vivo. BMC Neurosci. 10, 40 (2009).
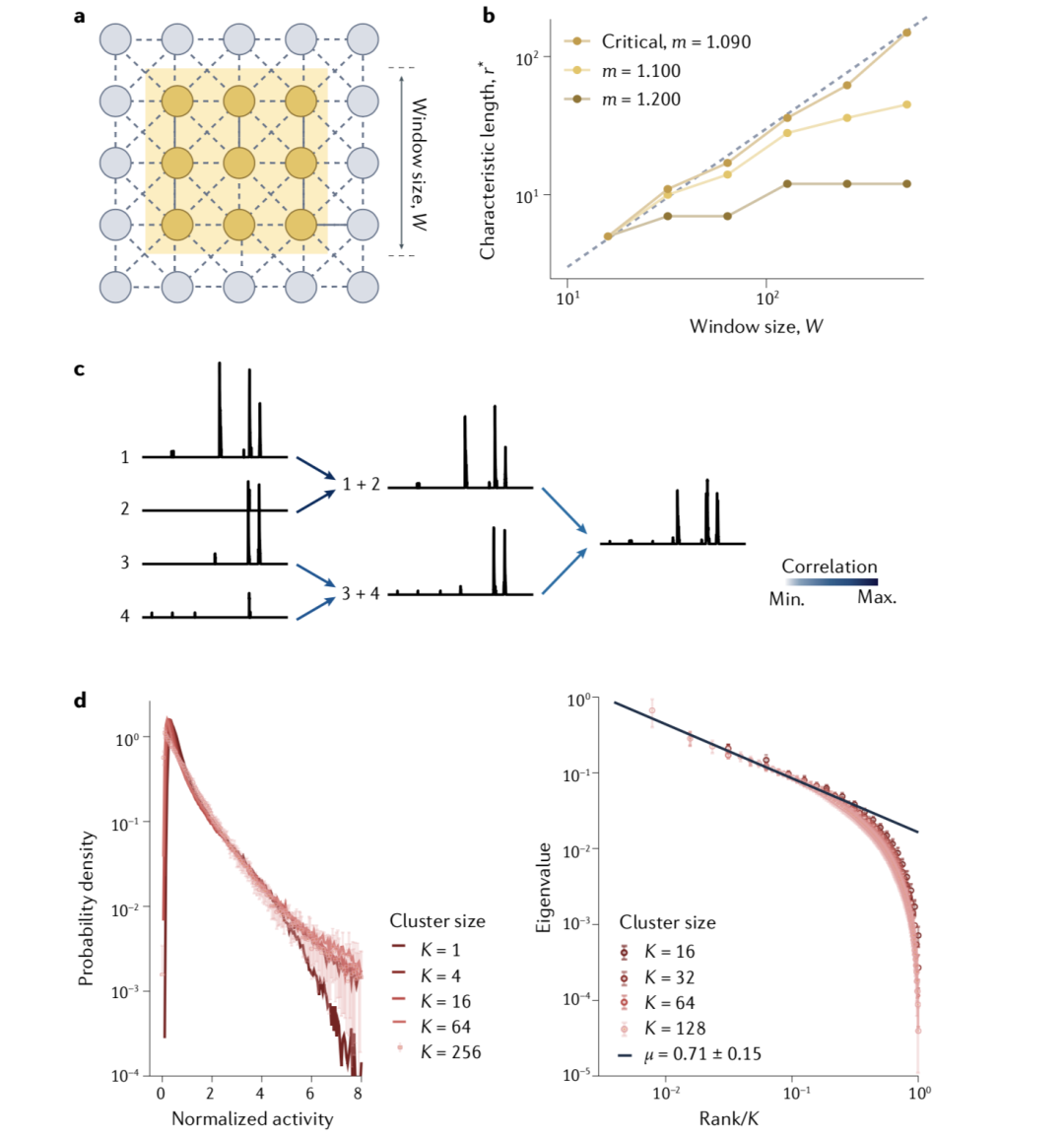
Magni, A., Durin, G., Zapperi, S. & Sethna, J. P. Visualization of avalanches in magnetic thin films: temporal processing. J. Stat. Mech. Theory Exp. 2009, P01020 (2009). 27. Chen, Y.-J., Papanikolaou, S., Sethna, J. P., Zapperi, S. & Durin, G. Avalanche spatial structure and multivariable scaling functions: sizes, heights, widths, and views through windows. Phys. Rev. E 84, 061103 (2011).
Zierenberg, J., Wilting, J., Priesemann, V. & Levina, A. Description of spreading dynamics by microscopic network models and macroscopic branching processes can differ due to coalescence. Phys. Rev. E 101, 022301 (2020).